| Admissions | Accreditation | A to Z Degree Fields | Booksellers | Catalog | Colleges | Contact Us | Continents/States/Districts | Contracts | Distance Education | Emergency | Examinations | Forms | Grants | Hostels | Honorary Doctorate degree | Human Services | Investment | Instructors | Login | Lecture | Librarians | Membership | Observers | Professional Examinations | Programs | Progress Report | Recommendations | Research Grants | Researchers | Students login | School | Search | Seminar | Study Center/Centre | Sponsorship | Tutoring | Thesis | Universities | Work counseling |
|
What is mathematics?
Mathematics is the study of quantity, structure, space, and change. What is the difference between math, maths, and mathematics? Math is a synonym of mathematics. Maths is a primarily _______ term for math. What are the subdivisions of mathematics? Mathematics can be subdivided broadly into the study of quantity, structure, space, and change (i.e., arithmetic, algebra, geometry, and mathematical analysis).
Where is math useful?
| ||||||||||||||||
| Alphabetical listing of Math topics | ||||||||||||||||
| Calculus | ||||||||||||||||
| Math for high school students | ||||||||||||||||
| Math for engineers | ||||||||||||||||
Mathematical analysis
Mathematical analysis looks at functions, sequences, and series. Mathematicians call mathematical analysis simply analysis. What are various subfields of mathematical analysis? | ||||||||||||||||
Math research
Existing math details are been discarded due to inability to answer relevant questions. New math facts are been circulated to enhance research, education, products, and services in essential ingredients of the economy. | ||||||||||||||||
|
Doctorate in Mathematics
Would you like to do a doctorate in mathematics? What should be the topic of your research? Here are further guidelines. |
Calculus
|
What is Calculus?
Calculus is the mathematical study of change, in the same way that geometry is the study of shape and algebra is the study of operations and their application to solving equations. It has two major branches, differential calculus (concerning rates of change and slopes of curves), and integral calculus (concerning accumulation of quantities and the areas under and between curves); these two branches are related to each other by the fundamental theorem of calculus. Here are further guidelines. |
Algebra
|
What is Algebra? Algebra is a branch of mathematics that substitutes letters for numbers, and an algebraic equation represents a scale where what is done on one side of the scale is also done to the other side of the scale and the numbers act as constants. Algebra can include real numbers, complex numbers, matrices, vectors, and many more forms of mathematic representation. College Preparatory Level: Elementary Algebra Intermediate Algebra College Level: College Algebra Linear Algebra Here are further guidelines. |
Excel in Basic Math, Algebra, Geometry, Trig, Calculus, Physics, Chemistry, Statistics, Programming, Electrical Engineering, Mechanical Engineering
Trigonometry
|
What is Trigonometry?
Trigonometry began as the computational component of geometry. • The tangent of angle A = the length of the opposite side divided by the length of the adjacent side • The sine of angle A = the length of the opposite side divided by the length of the hypotenuse • The cosine of angle A = the length of the adjacent side divided by the length of the hypotenuse
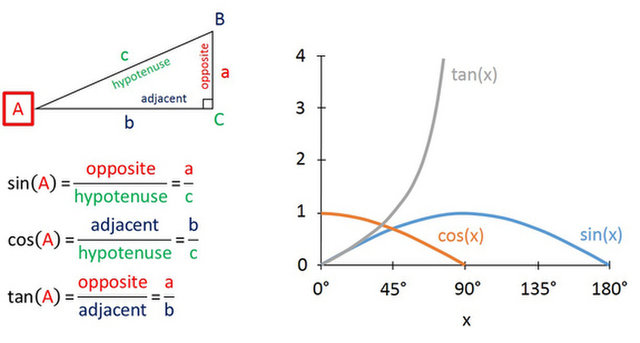Calculating trigonometric functions Today, scientific calculators have buttons for calculating the main trigonometric functions (sin, cos, tan, and sometimes cis and their inverses). Most allow a choice of angle measurement methods: degrees, radians, and sometimes gradians. Most computer programming languages provide function libraries that include the trigonometric functions. The floating point unit hardware incorporated into the microprocessor chips used in most personal computers has built-in instructions for calculating trigonometric functions. Applications There is an enormous number of uses of trigonometry and trigonometric functions. For instance, the technique of triangulation is used in astronomy to measure the distance to nearby stars, in geography to measure distances between landmarks, and in satellite navigation systems. The sine and cosine functions are fundamental to the theory of periodic functions, such as those that describe sound and light waves. Fields that use trigonometry or trigonometric functions include astronomy (especially for locating apparent positions of celestial objects, in which spherical trigonometry is essential) and hence navigation (on the oceans, in aircraft, and in space), music theory, audio synthesis, acoustics, optics, electronics, biology, medical imaging (CT scans and ultrasound), pharmacy, chemistry, number theory (and hence cryptology), seismology, meteorology, oceanography, many physical sciences, land surveying and geodesy, architecture, image compression, phonetics, economics, electrical engineering, mechanical engineering, civil engineering, computer graphics, cartography, crystallography and game development. Here are further guidelines. |